Cebeci–Smith model
The Cebeci–Smith model is a 0-equation eddy viscosity model used in computational fluid dynamics analysis of turbulent boundary layer flows. The model gives eddy viscosity, 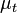 , as a function of the local boundary layer velocity profile. The model is suitable for high-speed flows with thin attached boundary-layers, typically present in aerospace applications. Like the Baldwin-Lomax model, this model is not suitable for cases with large separated regions and significant curvature/rotation effects. Unlike the Baldwin-Lomax model, this model requires the determination of a boundary layer edge.
, as a function of the local boundary layer velocity profile. The model is suitable for high-speed flows with thin attached boundary-layers, typically present in aerospace applications. Like the Baldwin-Lomax model, this model is not suitable for cases with large separated regions and significant curvature/rotation effects. Unlike the Baldwin-Lomax model, this model requires the determination of a boundary layer edge.
The model was developed by Tuncer Cebeci and Apollo M. O. Smith, in 1967.
Equations
In a two-layer model, the boundary layer is considered to comprise two layers: inner (close to the surface) and outer. The eddy viscosity is calculated separately for each layer and combined using:
where 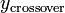 is the smallest distance from the surface where
is the smallest distance from the surface where 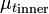 is equal to
is equal to 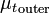 .
.
The inner-region eddy viscosity is given by:
where
with the von Karman constant  usually being taken as 0.4, and with
usually being taken as 0.4, and with
The eddy viscosity in the outer region is given by:
where 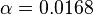 ,
, 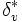 is the displacement thickness, given by
is the displacement thickness, given by
and FK is the Klebanoff intermittency function given by
References
- Smith, A.M.O. and Cebeci, T., 1967. Numerical solution of the turbulent boundary layer equations. Douglas aircraft division report DAC 33735
- Cebeci, T. and Smith, A.M.O., 1974. Analysis of turbulent boundary layers. Academic Press, ISBN 0-12-164650-5
- Wilcox, D.C., 1998. Turbulence Modeling for CFD. ISBN 1-928729-10-X, 2nd Ed., DCW Industries, Inc.
External links
- This article was based on the Cebeci Smith model article in CFD-Wiki
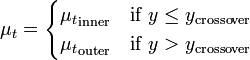
![{\mu_t}_\text{inner} = \rho \ell^2 \left[\left(
\frac{\partial U}{\partial y}\right)^2 +
\left(\frac{\partial V}{\partial x}\right)^2
\right]^{1/2}](../I/m/bbacb2b389572733404bdc3561680360.png)
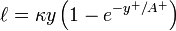
![A^+ = 26\left[1+y\frac{dP/dx}{\rho u_\tau^2}\right]^{-1/2}](../I/m/28a902f9e8999378da20ff20b304aaf1.png)
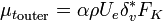
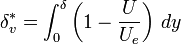
![F_K = \left[1 + 5.5 \left( \frac{y}{\delta} \right)^6
\right]^{-1}](../I/m/5382b742eb2a9c7d54d2f728303fc595.png)