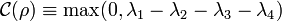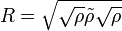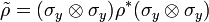Concurrence (quantum computing)
In quantum information science, the concurrence is a state invariant involving qubits.
Definition
the concurrence is an entanglement monotone defined for a mixed state of two qubits as:[1][2][3][4]
in which 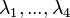 are the eigenvalues, in decreasing order, of the Hermitian matrix
are the eigenvalues, in decreasing order, of the Hermitian matrix
with
the spin-flipped state of 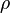 ,
, 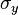 a Pauli spin matrix, and the eigenvalues listed in decreasing order.
a Pauli spin matrix, and the eigenvalues listed in decreasing order.
Other formulations
Alternatively, the 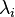 's represent the square roots of the eigenvalues of the non-Hermitian matrix
's represent the square roots of the eigenvalues of the non-Hermitian matrix 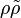 .[2] Note that each
.[2] Note that each 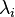 is a non-negative real number. From the concurrence, the entanglement of formation can be calculated.
is a non-negative real number. From the concurrence, the entanglement of formation can be calculated.
Properties
For pure states, the concurrence is a polynomial 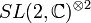 invariant in the state's coefficients.[5] For mixed states, the concurrence can be defined by convex roof extension.[3]
invariant in the state's coefficients.[5] For mixed states, the concurrence can be defined by convex roof extension.[3]
For the concurrence, there is monogamy of entanglement,[6][7] that is, the concurrence of a qubit with the rest of the system cannot ever exceed the sum of the concurrences of qubit pairs which it is part of.
References
- ↑ Scott Hill and William K. Wootters, Entanglement of a Pair of Quantum Bits, 1997.
- 1 2 William K. Wootters, Entanglement of Formation of an Arbitrary State of Two Qubits 1998.
- 1 2 Roland Hildebrand, Concurrence revisited, 2007
- ↑ Ryszard Horodecki, Paweł Horodecki, Michał Horodecki, Karol Horodecki, Quantum entanglement, 2009
- ↑ D. Ž. Ðoković and A. Osterloh, On polynomial invariants of several qubits, 2009
- ↑ Valerie Coffman, Joydip Kundu, and William K. Wootters, Distributed entanglement, 2000
- ↑ Tobias J. Osborne and Frank Verstraete, General Monogamy Inequality for Bipartite Qubit Entanglement, 2006