Conjugate index

In mathematics, two real numbers 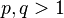$p, q>1$ are called conjugate indices if

$\frac{1}{p} + \frac{1}{q} = 1.$

Formally, we will also define 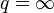$q = \infty$ as conjugate to 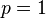$p=1$ and vice versa.

Conjugate indices are used in Hölder's inequality. Also, if 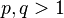$p, q>1$ are conjugate indices, the spaces L^p and L^q are dual to each other (see L^p space).

See also

• Beatty's theorem

References

• A B Antonevich, Linear Functional Equations, Birkhäuser, 1999. ISBN 3-7643-2931-9.

This article incorporates material from Conjugate index on PlanetMath, which is licensed under the Creative Commons Attribution/Share-Alike License.