Gian Francesco Malfatti

Gian Francesco Malfatti.

Giovanni Francesco Giuseppe, also known as Gian Francesco or Gianfrancesco (26 September 1731 – 9 October 1807) was an Italian mathematician. He was born in Ala, Trentino, Italy and died in Ferrara.

Malfatti studied at the College of San Francesco Saverio in Bologna where his mentors included Vincenzo Riccati, F. M. Zanotti and Gabriele Manfredi. He moved to Ferrara in 1754, and became a professor at the University of Ferrara when it was re-established in 1771. In 1782 he was one of the founders of the Societa Italiana delle Scienze, later to become the Accademia nazionale delle scienze detta dei XL.

Malfatti circles

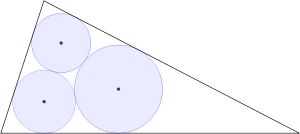
Malfatti posed the problem of carving three circular columns out of a triangular block of marble, using as much of the marble as possible, and conjectured that three mutually-tangent circles inscribed within the triangle would provide the optimal solution. These tangent circles are now known as Malfatti circles after his work, despite the earlier work of Japanese mathematician Ajima Naonobu and of Malfatti's countryman Gilio di Cecco da Montepulciano on the same problem[1][2] and despite the fact that the conjecture was later proven false.[3] Several triangle centers derived from these circles are also named after both Ajima and Malfatti.[4][5]

The gravitational property of the lemniscate

Additional topics in Malfatti's research concerned quintic equations, and the property of the lemniscate of Bernoulli that a ball rolling down an arc of the lemniscate, under the influence of gravity, will take the same time to traverse it as a ball rolling down a straight line segment connecting the endpoints of the arc.

Notes

  1. ↑ Fukagawa, Hidetoshi; Rothman, Tony (2008), Sacred mathematics: Japanese temple geometry, Princeton University Press, p. 79, ISBN 978-0-691-12745-3.
  2. ↑ Simi, A.; Toti Rigatelli, L. (1993), "Some 14th- and 15th-century texts on practical geometry", Vestigia mathematica, Amsterdam: Rodopi, pp. 453–470, MR 1258835.
  3. ↑ Goldberg, M. (1967), "On the Original Malfatti Problem", Mathematics Magazine, 40: 241–247, JSTOR 2688277.
  4. ↑ Weisstein, Eric W. "Ajima-Malfatti Points". MathWorld. .
  5. ↑ C. Kimberling, Encyclopedia of Triangle Centers, X(179), X(180), and X(400).

References

External links

This article is issued from Wikipedia - version of the 9/19/2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.