Pretzel link

In the mathematical theory of knots, a pretzel link is a special kind of link. A pretzel link which is also a knot (i.e. a link with one component) is a pretzel knot.
In the standard projection of the pretzel link, there are left-handed crossings in the first tangle, in the second, and, in general, in the nth.
A pretzel link can also be described as a Montesinos link with integer tangles.
Some basic results
The pretzel link is a knot iff both and all the are odd or exactly one of the is even.[1]
The pretzel link is split if at least two of the are zero; but the converse is false.
The pretzel link is the mirror image of the pretzel link.
The pretzel link is link-equivalent (i.e. homotopy-equivalent in S3) to the pretzel link. Thus, too, the pretzel link is link-equivalent to the pretzel link.[1]
The pretzel link is link-equivalent to the pretzel link. However, if one orients the links in a canonical way, then these two links have opposite orientations.
Some examples
The (1, 1, 1) pretzel knot is the (right-handed) trefoil; the (−1, −1, −1) pretzel knot is its mirror image.
The (5, −1, −1) pretzel knot is the stevedore knot (61).
If p, q, r are distinct odd integers greater than 1, then the (p, q, r) pretzel knot is a non-invertible knot.
The (2p, 2q, 2r) pretzel link is a link formed by three linked unknots.
The (−3, 0, −3) pretzel knot (square knot (mathematics)) is the connected sum of two trefoil knots.
The (0, q, 0) pretzel link is the split union of an unknot and another knot.
Montesinos
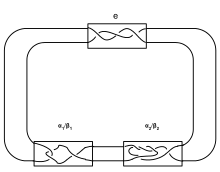
A Montesinos link is a special kind of link that generalizes pretzel links (a pretzel link can also be described as a Montesinos link with integer tangles). A Montesinos link which is also a knot (i.e. a link with one component) is a Montesinos knot.
A Montesinos link is composed of several rational tangles. One notation for a Montesinos link is .[2]
In this notation, and all the and are integers. The Montesinos link given by this notation consists of the sum of the rational tangles given by the integer and the rational tangles
Utility

(−2, 3, 2n + 1) pretzel links are especially useful in the study of 3-manifolds. Many results have been stated about the manifolds that result from Dehn surgery on the (−2,3,7) pretzel knot in particular.
The hyperbolic volume of the complement of the (−2,3,8) pretzel link is 4 times Catalan's constant, approximately 3.66. This pretzel link complement is one of two two-cusped hyperbolic manifolds with the minimum possible volume, the other being the complement of the Whitehead link.[3]
References
- 1 2 Kawauchi, Akio (1996). A survey of knot theory. Birkhäuser. ISBN 3-7643-5124-1
- ↑ Zieschang, Heiner. "Classification of Montesinos knots." Topology. Springer Berlin Heidelberg, 1984. 378–389
- ↑ Agol, Ian (2010), "The minimal volume orientable hyperbolic 2-cusped 3-manifolds", Proceedings of the American Mathematical Society, 138 (10): 3723–3732, doi:10.1090/S0002-9939-10-10364-5, MR 2661571.
Further reading
- Trotter, Hale F.: Non-invertible knots exist, Topology, 2 (1963), 272–280.
- Burde, Gerhard; Zieschang, Heiner (2003). Knots. De Gruyter studies in mathematics. 5 (2nd revised and extended ed.). Walter de Gruyter. ISBN 3110170051. ISSN 0179-0986. Zbl 1009.57003.