Simpson correspondence

In algebraic geometry, the Nonabelian Hodge correspondence or Corlette-Simpson correspondence (named after Kevin Corlette and Carlos Simpson) is a correspondence between Higgs bundles and representations of the fundamental group.

Unitary representations

For a compact Riemann surface X of genus at least 2, Narasimhan and Seshadri established a bijection between irreducible unitary representations of 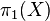$\pi_1(X)$ and the isomorphism classes of stable vector bundles of degree 0.^[1] This was then extended to arbitrary complex smooth projective varieties by Uhlenbeck-Yau and by Donaldson.^[2]

Arbitrary representations

These results were extended to arbitrary rank two complex representations of fundamental groups of curves by Hitchin ^[3] and Donaldson ^[4] and then in arbitrary dimension by Corlette ^[5] and Simpson,^[6][7] who established an equivalence of categories between the finite-dimensional complex representations of the fundamental group and the semi-stable Higgs bundles whose Chern class is zero.

References

1. M.S. Narasimhan, C.S. Seshadri, Stable and unitary vector bundles on a compact Riemann surface, Ann. of Math. 82 (1965), 540–567
2. S. Donaldson, Infinite determinants, stable bundles and curvature, Duke Math. J. 54 (1987), 231–247
3. N. J. Hitchin, The self-duality equations on a Riemann surface Proceedings of the London Mathematical Society. 55 no.1 1987, 59–126
4. S. K. Donaldson Twisted harmonic maps and the self-duality equations Proceedings of the London Mathematical Society. 55 no.1 1987, 127–131
5. K. Corlette, Flat G-bundles with canonical metrics J. Differential Geometry 28 no. 3 1988 361–382
6. C. T. Simpson, Nonabelian Hodge Theory, ICM talk, Kyoto 1990, http://ada00.math.uni-bielefeld.de/ICM/ICM1990.1/Main/icm1990.1.0747.0756.ocr.pdf
7. C. T. Simpson, Higgs bundles and local systems. Inst. Hautes Études Sci. Publ. Math. No. 75 (1992), 5–95