Taylor column

Diagram of a Taylor column

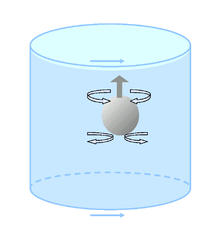
A Taylor column is a feature of the coriolis effect. It was named after Geoffrey Ingram Taylor. Rotating fluids that are perturbed tend to form columns parallel to the axis of rotation called Taylor columns.

In a rotating fluid a strongly buoyant ball (such as a pingpong ball) will rise to the surface slower than it would in a non rotating fluid. Fluid that is shifted from its position in the rotating system tends to be pushed back to the point it is shifted away from, the faster the rotation rate, the smaller the radius of the inertial circle.

A unit of fluid (represented by the black dot) is pushed back to the point it is shifted from.

In a non-rotating fluid the fluid parts above the rising ball and closes in underneath it, offering relatively little resistance to the ball. In a rotating fluid, if certain conditions are met, the ball needs to push up a whole column of fluid above it, and it needs to drag a whole column of fluid along beneath it in order to rise to the surface.

A rotating fluid thus displays some degree of rigidity, and no longer behaves entirely like a fluid anymore.

History

Taylor columns were first observed by William Thomson, Lord Kelvin, in 1868.[1][2] Taylor columns were featured in lecture demonstrations by Kelvin in 1881[3] and by John Perry in 1890.[4] The phenomenon is explained via the Taylor-Proudman theorem, and it has been investigated by Taylor,[5] Grace,[6] Stewartson,[7] and Maxworthy[8] — among others.

References

  1. ↑ James Thomson (Lord Kelvin's brother) (1868) Letter to William Thomson (30 September). Glasgow University Library, MS Kelvin T120.
  2. ↑ O. U. Velasco Fuentes (2008) "Kelvin’s discovery of Taylor columns," European Journal of Mechanics - B / Fluids, vol. 28, no. 3, pages 469-472 (May-June 2008). Available on-line at: http://oceanografia.cicese.mx/oscar/pdf/VF2009.pdf
  3. ↑ Thomson, W. (1882) "Elasticity viewed as possibly a mode of motion," Proceedings of the Royal Institution, vol. 9, pages 520–521; also in: Popular Lectures and Addresses, vol. 1, pages 142–146. See also: Thomson, W. (1887) "On the stability of steady and of periodic fluid motion," Philosophical Magazine, vol. 23, pages 459–464. Also in: Mathematical and Physical Papers (Cambridge, England: Cambridge University Press, 1910), vol. 4, pp. 166-172.
  4. ↑ Perry, J. Spinning tops. The “Operatives’ lecture” of the British Association meeting at Leeds, 6th September, 1890. (London: Society for Promoting Christian Knowledge, 1910).
  5. ↑ Taylor, G.I. (1922) "The motion of a sphere in a rotating liquid," Proceedings of the Royal Society of London A, vol. 102, pages 180–189.
  6. ↑ Grace, S.F. (1922) "Free motion of a sphere in a rotating liquid parallel to the axis of rotation," Proceedings of the Royal Society of London A, vol. 102, pages 89–111.
  7. ↑ Stewartson, K. (1952) "On the slow motion of a sphere along the axis of a rotating fluid," Proceedings of the Cambridge Philosophical Society, vol. 48, pages 168–177.
  8. ↑ Maxworthy, T. (1968) "The observed motion of a sphere through a short, rotating cylinder of fluid," Journal of Fluid Mechanics, vol. 31, pages 643–655. See also: Maxworthy, T. (1970) "The flow created by a sphere moving along the axis of a rotating, slightly-viscous fluid," Journal of Fluid Mechanics, vol. 40, pages 453–479.

External links

This article is issued from Wikipedia - version of the 11/29/2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.