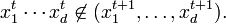Monomial conjecture
In commutative algebra, a field of mathematics, the monomial conjecture of Melvin Hochster says the following:[1]
Let A be a Noetherian local ring of Krull dimension d and let x1, ..., xd be a system of parameters for A (so that A/(x1, ..., xd) is an Artinian ring. Then for all positive integers t, we have
The statement can relatively easily be shown in characteristic zero.
References
This article is issued from Wikipedia - version of the 3/29/2014. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.