Sailing faster than the wind

Some wind-powered vehicles such as sailboats, iceboats and sand yachts are able to advance over the surface on which they travel faster than the wind that powers them.^[1] Such devices cannot do this when sailing dead downwind using simple square sails that are set perpendicular to the wind, but they can achieve speeds greater than wind speed by setting sails at an angle to the wind, or employing propellers, and by using the lateral resistance of the surface on which they sail (for example the water or the ice).^{[2][3][4][5][6][7]}

In 2013, a new class of catamaran was announced for the America's Cup which can achieve well in excess of double the speed of the wind.^[8] The Extreme 40 catamaran can sail at 35 knots (65 km/h; 40 mph) in 20–25-knot (37–46 km/h; 23–29 mph) winds.^[9] The high-performance International C-Class Catamaran can sail at twice the speed of the wind.^[10] Iceboats can typically sail at five times the speed of the wind.^[11] By sailing downwind at 135 degrees off the wind, a sand yacht can sail much faster than the wind.^[12] The velocity made good downwind is often over twice as fast compared to the same land yacht sailing directly downwind.^[12] The catamarans used for the 2013 America's Cup were expected to sail upwind at 1.2 times the speed of the true wind, and downwind at 1.6 times the speed of the true wind.^[13][14][15] They proved to be faster, averaging about 1.8 times the speed of the wind with peaks slightly over 2.0.^[16]

In 2009, the world speed sailing record on water was set by a hydrofoil trimaran sailing at 1.71 times the speed of the wind.^[17][18] Also in 2009, the world land speed record for a wind-powered vehicle was set by the sand yacht Greenbird sailing at about three times the speed of the wind.^[19] In late 2012 the Vestas Sailrocket 2 skippered by Paul Larsen achieved a new outright world speed record of 65.45 knots on water, at around 2.5 times the speed of the wind.^[20]

Sailing perpendicular to the wind

For example, a boat can sail a course that is perpendicular to the true wind (that is, at 90 degrees with respect to the true wind). As it accelerates, the wind as seen from the boat will increase and the wind will appear to shift forward.^[21] This is the same effect that causes rain to appear to fall at an angle when seen from a moving car, and is analogous to the astronomical phenomenon of aberration of light.

As the wind increases in speed and shifts forward (because of the acceleration of the boat), the sails have to be trimmed in order to maintain performance. This causes the boat to further accelerate, thus causing a further increase in windspeed and a further forward windshift.

Eventually, the sails cannot be trimmed any further and an equilibrium is reached. Although the boat is sailing perpendicular to the true wind, its sails are set for close hauled sailing.^[22]

The actual speed of the boat in such a situation depends on the wind speed, how close to the wind it can sail, the resistance of the surface (water or ice), and leeway (downwind drift). Normal cruising boats yachts can sail at about 45 degrees off the apparent wind (50 to 60 degrees off the true wind). High performance racing yachts at about 27 degrees (35 degrees off the true wind).^[23] High-performance multihulls can sail at 20 degrees off the apparent wind.^[24] Iceboats can sail even closer to the apparent wind. According to the data provided on p. 406 of the cited book High Performance Sailing, a fast keelboat such as a Soling can sail at 30 degrees off the apparent wind, an 18ft Skiff at 20 degrees, and an iceboat at 7 degrees.^[25]

If hull speed is not a limiting factor, and if the strength of the wind is sufficient to overcome the surface resistance, then the speed of the boat as a multiple of the wind speed will depend only on how close it can sail to the wind. For example, assuming that surface resistance is negligible (as for an iceboat), if a boat sails at 90 degrees to the true wind, but at 45 degrees to the apparent wind, then it must be sailing at the same speed as the true wind. That is, if the wind speed is V, then the boat's speed is also V. Elementary trigonometry and elementary vector operations can be used to show that, if a boat sails at 90 degrees to the true wind, but at alpha degrees to the apparent wind, and the wind speed is V, then the boat's speed must be V×cotangent(alpha). The table below shows the values of this function, as a multiple of windspeed.

Alpha	Multiple of windspeed
45	1.00
40	1.19
35	1.43
30	1.73
25	2.14
20	2.75
15	3.73
10	5.67

Hull speed is not a limiting factor for an iceboat nor for high-performance multihulls. So a boat capable of sailing at 10 degrees off the apparent wind (which is the case for many iceboats) that sails at 90 degrees to the true wind will be sailing nearly 6 times faster than the wind.^[26] It can sail slightly faster, as a multiple of the windspeed, if it sails at a greater angle off the true wind.^[27]

Sailing on a broad reach

As stated in the Introduction of the book High Performance Sailing,^[5] in the section Tacking Downwind, "any boat which runs 'square' must necessarily sail downwind at some speed less than the wind's speed whereas any boat which tacks downwind has no theoretical limit to its speed. Ice yachts, for example, can tack downwind at average speeds many times the wind speed."

The same book states, in section 24.2, "In a True Wind of 15 knots (28 km/h; 17 mph), the Soling crew will sail the close reach and reaching legs in Apparent Winds little stronger than True Wind. ... The 18-foot Skiff crew sails the cross wind legs in much stronger Apparent Winds which approach 30 knots (56 km/h; 35 mph). Even on the broad reaching legs they must still sail in a strong Apparent Wind which blows from ahead, so they still need to use strong-wind 'going-to-windward' handling techniques even though they are sailing downwind." Figure 24.2 of the book provides vector graphics that show how the 18-foot (5.5 m) Skiff can sail downwind faster than the speed of the wind.

From the detailed data provided for the 2009 record set by a sand yacht, it can be seen that the record was achieved when the yacht's course was about 120 degrees off the true wind.^[28] That is, the yacht was moving faster than the wind although the true wind was behind it. This is possible because the speed of the yacht results in a large forward wind shift, so that the yacht is close hauled with respect to the apparent wind.^[29]

Suppose that a boat is at a standstill, then starts to sail on a course that is 135 degrees off the true wind (the value 135 is chosen for this explanation in order to simplify certain calculations). The boat will accelerate, so the apparent wind will be less than the true wind and will shift forward of the true wind. If the boat can reach a speed equal to about 71% of the true windspeed, then the apparent wind will be perpendicular to the boat's course and its speed will also be about 71% of the true windspeed. If that reduced apparent windspeed still generates sufficient force to overcome the resistance of the surface, then the boat will further accelerate.

That is, the situation will be the same as the one explained above, since the boat is now accelerating after having reached a course perpendicular to the apparent wind. In practice, most boats sailing on the water cannot overcome the resistance of the water in order to reach speeds equal to the speed of the wind. However, iceboats can do so, because the resistance of the surface is very small. Thus, an iceboat that starts sailing on a broad reach will continue to accelerate until it is close-hauled with respect to the apparent wind.^[30]

The table^[31] and diagram below illustrate this situation. The vector labelled "boat speed" represents the relative wind resulting from the boat's progress through the water, that is, the wind that is induced by the boat's motion: its speed is the same as the speed of the boat and its direction is directly opposite to the direction of the boat's motion. Both boat speed and apparent wind speed are shown as a fraction (or multiple) of true wind speed, which is represented by the vertical vector at the left of the diagram.

Boat speed	Alpha	Apparent wind speed
0.10	131	0.93
0.30	120	0.82
0.50	106	0.74
0.71	90	0.71
1.00	68	0.77
1.41	45	1.00
2.00	29	1.47
3.00	17	2.40
4.00	12	3.37
5.00	9	4.35

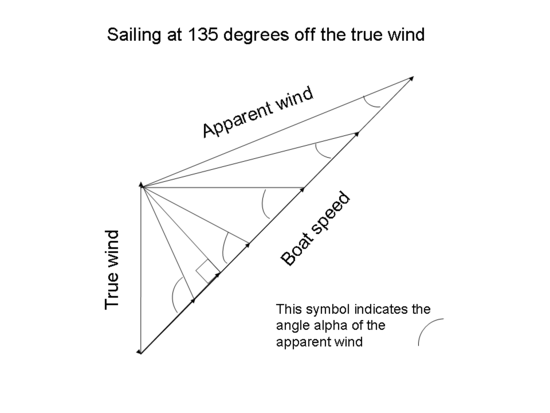
Note that, if a boat can accelerate until it is sailing at 45 degrees off the apparent wind when sailing 135 degrees off the true wind, then its speed will be 1.41 times the speed of the true wind. Thus its velocity made good downwind will be equal to the velocity of the true wind. If it can accelerate until it is sailing closer than 45 degrees to the apparent wind, then its velocity made good downwind will be greater than the velocity of the true wind: see the more detailed discussion in the section Speed made good below.

Vector diagrams and formulas

As explained in the article on apparent wind, a boat's forward motion creates a corresponding head wind of the same strength in the opposite direction. That head wind must be combined with the true wind to find the apparent wind.^[32]

The drawing below shows the vector operations and resulting calculations for sailing upwind. Alpha is the angle of the apparent wind. Beta is the course of the boat with respect to the true wind. The true wind is assumed to be equal to 1 in order to simplify the formulas. Note that the true wind is added using vector addition to the head wind created by the boat's speed.

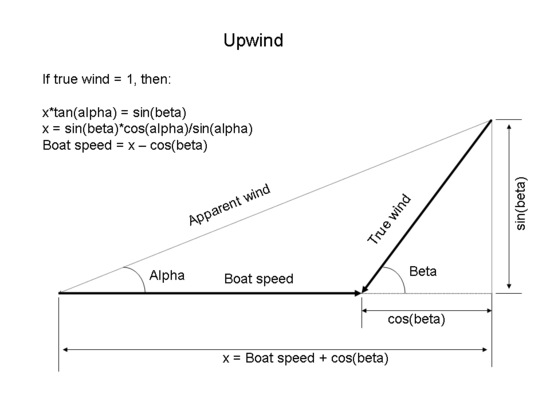
The drawing below shows the vector operations and resulting calculations for sailing downwind. Alpha is the angle of the sails to the apparent wind. Beta is the course of the boat with respect to the true wind. The true wind is assumed to be equal to 1 in order to simplify the formulas. Note that the true wind is added to the head wind created by the boat's speed.

The drawing below shows apparent wind angles and speeds for different boat speeds for a boat sailing downwind at 135 degrees.

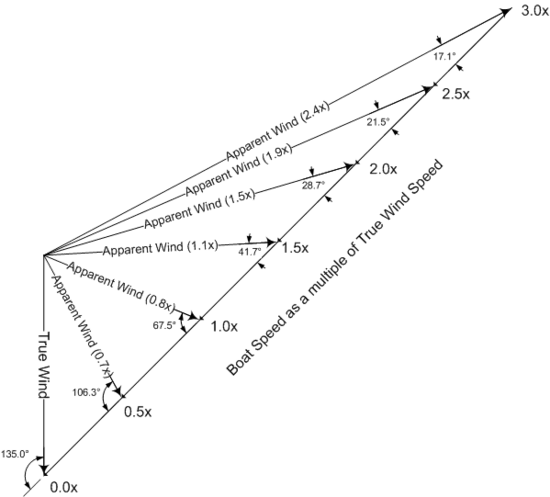
Note that for both the upwind and downwind cases, the apparent crosswind (the component of true wind perpendicular to the heading of a boat) is true wind speed x sin(Beta), independent of the boat's speed. The maximum speed of a sail boat is a function of how fast it can travel for a given apparent crosswind and the drag from the water (or land or ice) related to the boat's true speed. Since the drag from the water (or land or ice) increases with the boat's true speed, the boat achieves it's maximum apparent wind on an upwind tack, where it's true speed is the slowest. This is why minimum beta for a boat is smaller upwind than it is downwind.

The Beta Theorem and Maximum speed course sailing angle

Beta Theorem

Ross Garrett describes the Beta Theorem on page 67 in his book "The Symmetry of Sailing". The Beta Theorem is otherwise known as the Course Theorem and is also described here Course Theorem.

Simply stated, consider the two drag angles - the hull (below-water) drag angle and rig (above-water) drag angle - of a yacht. The sum of those two drag angles are equal to the apparent wind angle (the angle between the apparent wind and the course sailed).

The drag angles are composite, and are found from the inverse trigonometrical tangent of the lift to drag ratios. For the hull, the drag angle is complicated because there are several drags .. for example - you would first take the total drag of the keel-centreboard-fin and the hull, and divide this by the total sideways force (i.e. this matches the sail's sideways force) - and then take the inverse tan of this ratio. Similarly for the rig (sails, stays etc.) drag angle.

The Beta theorem always applies to a yacht, no matter what direction it is sailing, and no matter what the sheeting angle (yes even luffing). This latter point illustrates that the Beta theorem must use the actual current drag angles, not the optimum ones of the craft. Ross Garrett emphasizes this point.

The efficiency of a yacht can clearly be understood in terms of the Beta Theorem. When the lift to drag ratios of the hull and the rig are both very good (high), then the angle Beta will be low, and correspondingly the apparent wind angle will be low, and the yacht can and will be sailing faster than the wind on many different headings.

Maximum speed course sailing angle

"A yacht which can sail faster than the wind can sail at its maximum efficiency (ratio of yacht speed to true wind speed) only when the apparent wind and the true wind are at right angles."

This is stating the potential for efficiency, and is especially applicable to speed sailing efforts, where the craft is tuned (e.g. reefed down or up, or bigger fin used etc.) for the chosen angle of each speed run.

The maximum speed course sailing angle conclusion is shown to be a corollary of the Beta Theorem, with the assistance of Figures 1-3.

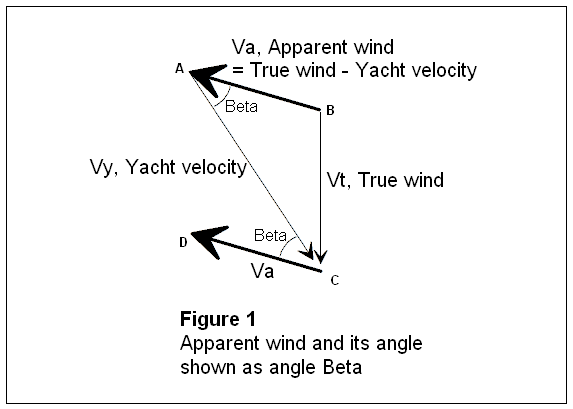
Figure 1 shows the relationship of the true wind and apparent wind vectors as differing by the yacht velocity. Also shown is the angle Beta, which is the "apparent wind angle" i.e. the angle between the yacht heading and the apparent wind on the yacht.

Beta is assumed to be constant. In practice this is not perfectly true. However, in the quest for maximum speed, it can be made to be true .. i.e. it is assumed that the size of the yacht sails and fin or centreboard are chosen for each speed run so as to maintain the same (optimal) operating points, and therefore the same lift to drag ratios, and correspondingly a constant Beta for each different heading under test.

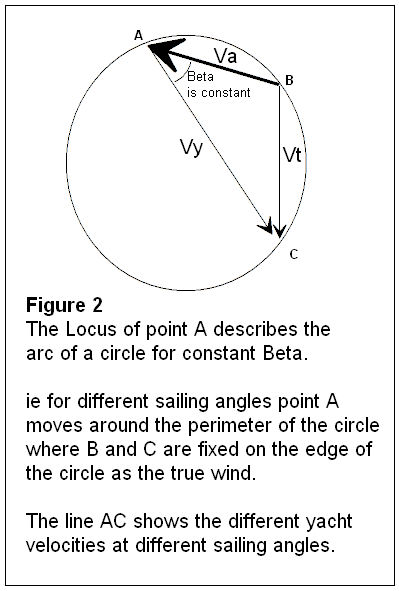
Having accepted the constraints for a constant Beta, then it is simple maths (a constant angle is subtended on the arc of a circle) that we arrive with the locus of a circle for one end of the vector showing yacht velocity, as in Figure 2.

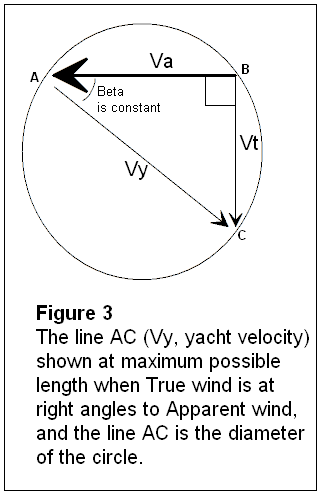
Now the maximum yacht velocity clearly will occur when the vector Vy lies across the diameter of the circle, as shown in Figure 3. In this figure, the apparent wind and the true wind are at right angles. Also note that the optimum speed sailing angle is equal to the apparent wind angle (Beta) below a beam reach.

Vt and Va appear equal in figure 3, where Vy is about 1.4x Vt. This is not the general case. The more efficient the yacht, the smaller will be Beta, and the closer to a beam reach will be the optimum speed sailing course. The ultimate ratio of yacht speed to wind speed therefore also gives rise to Beta by inverse cosine. e.g. speed-sailing at twice the windspeed corresponds to a 30 degrees apparent wind angle i.e. Beta = 30 degrees.

Speed sailing windsurfers have an apparent wind angle somewhat worse (greater) than 30 degrees, and accordingly their speed sailing courses are set for a broader sailing angle than this. Speed sailing kite boards prefer an even broader course than windsurfers. By contrast, the highly efficient Vestas Sailrocket has a design Beta of about 20 degrees (the hull is offset this amount from the centreline) and this is how close to a beam reach she may have been sailing.

Speed made good

Most sailing is not done in order to achieve a maximum speed, but in order to go from one point to another. In most sailboat racing the objective is to sail a certain distance directly upwind (to a point called the upwind mark), and then to return downwind, as fast as possible.

Since sailboats cannot sail directly into the wind, they must tack in order to reach the upwind mark (this process is called beating or working to the mark). This lengthens the course, thus the boat takes longer to reach the upwind mark than it would if it could have sailed directly towards it. The component of a sailboat's speed that is in the direction of the next mark is called the velocity made good.^[33]

If a boat sails perpendicular to the wind, it will never reach the upwind mark. So, in racing, speed is not everything. What counts is the velocity made good, that is, the progress towards the upwind mark. Again, simple trigonometry can be used to calculate the velocity made good. The tables below show velocity made good, again as a multiple of windspeed, and again assuming negligible surface resistance. The first column indicates the course as an angle off the true wind. Alpha is again the closest angle to the apparent wind at which the boat can sail. The calculation assumes that the boat accelerates until the apparent wind is alpha degrees off the bow.

Speed made good as multiple of windspeed

Alpha Upwind Course 10 15 20 25 30 35 40 45 10 20 0.94 0.32 30 1.71 0.87 0.44 0.18 40 2.21 1.25 0.77 0.47 0.27 0.12 50 2.38 1.42 0.94 0.64 0.44 0.29 0.17 0.08 60 2.21 1.37 0.94 0.68 0.50 0.37 0.27 0.18 70 1.71 1.08 0.77 0.57 0.44 0.34 0.27 0.20 80 0.94 0.61 0.44 0.34 0.27 0.21 0.17 0.14 90 0.00 0.00 0.00 0.00 0.00 0.00 0.00 0.00

Alpha Downwind Course 10 15 20 25 30 35 40 45 100 1.00 0.67 0.50 0.40 0.33 0.27 0.23 0.20 110 1.94 1.32 1.00 0.81 0.67 0.58 0.50 0.44 120 2.71 1.87 1.44 1.18 1.00 0.87 0.77 0.68 130 3.21 2.25 1.77 1.47 1.27 1.12 1.00 0.91 140 3.38 2.42 1.94 1.64 1.44 1.29 1.17 1.08 150 3.21 2.37 1.94 1.68 1.50 1.37 1.27 1.18 160 2.71 2.08 1.77 1.57 1.44 1.34 1.27 1.20 170 1.94 1.61 1.44 1.34 1.27 1.21 1.17 1.14 180 1.00 1.00 1.00 1.00 1.00 1.00 1.00 1.00

It can be seen that a boat that can sail closer than 20 degrees to the apparent wind can make good upwind faster than the real wind.

Many boats can make good downwind faster by not sailing dead downwind, but instead jibing (also spelled gybing) back and forth.^[34] If the boat can accelerate until the apparent wind is alpha degrees off the bow, then it can be seen from the table above that it can make good downwind faster than the true wind.^[35] Such performance is theoretically possible.^[36] An easy-to-grasp animation demonstrating the principle of how it can be possible to go faster than the wind can be found at.^[37]

However real boats cannot equal the performances shown in the table,^[38] although iceboats can come close to them. Indeed iceboats can make good both upwind and downwind at speeds greater than the wind.^[39][40] And so can sand yachts: during the 2009 land speed record, the yacht Greenbird was proceeding at about 3 times the speed of the wind on a course about 120 degrees off the true wind.^[19] Thus, its speed made good downwind was about 1.5 times the speed of the wind. During a training run the catamaran Alinghi 5, one of the competitors for the 2010 America's Cup, covered 20 nautical miles (37 km; 23 mi) to windward and back in 2.5 hours in 8–9-knot (15–17 km/h; 9.2–10.4 mph) winds, so its average velocity made good was 16 knots (30 km/h; 18 mph), about 1.9 times wind speed.^[41] This is consistent with the yacht being able to sail at about 15 degrees off the apparent wind, see the table above. Indeed, the catamaran sails so fast downwind that the apparent wind it generates is only 5-6 degrees different from that when it is racing upwind; that is, the boat is always sailing upwind with respect to the apparent wind.^[42]

During the first race of the 2010 America's Cup, the winning yacht USA 17 sailed 20 nautical miles (37 km; 23 mi) to windward in 1 hour 29 minutes, in winds of 5–10 knots (9.3–18.5 km/h; 5.8–11.5 mph). Thus its velocity made good upwind was about 1.8 times windspeed, consistent with being able to sail about 13 degrees off the apparent wind when sailing upwind. She sailed 20 nautical miles (37 km; 23 mi) downwind in 1 hour 3 minutes, so her velocity made good downwind was about 2.5 times windspeed, consistent with being able to sail about 14 degrees off the apparent wind when sailing downwind.^{[43][44][45][46]} During the second race, winds were 7–8 knots (13–15 km/h; 8.1–9.2 mph). USA 17 reached the windward mark in 59 minutes, so her velocity made good was about 13.2 knots (24.4 km/h; 15.2 mph), about 1.65 times wind speed. The course was a triangle, so the velocity made good downwind was only 11.5 knots (21.3 km/h; 13.2 mph), about 1.4 times wind speed. USA 17 averaged 26.8 knots (49.6 km/h; 30.8 mph), about 3.35 times the wind speed, on the faster first triangular leg.^[47][48][49]

Other sailboats (such as the 18ft Skiff) can make good downwind at speeds faster than the wind.^[50] Indeed, it can be seen from the polar chart^[51] for the 18 ft (5.5 m) Skiff that it can make good about 12 knots (22 km/h; 14 mph) downwind at a windspeed of 10 knots (19 km/h; 12 mph), by jibing back and forth at about 140 degrees off the true wind.^[50][52][53] The polar chart in Figure PS1 of the cited book High Performance Sailing^[5] shows that boats that were sailing in 1996 were able to make good downwind at about 1.5 times the speed of the wind.

Directly down wind faster than the wind (DDWFTTW) vehicle

It is possible to create a vehicle that moves directly downwind faster than the wind by having its wheels drive a propeller against the wind. The propeller slows down the wind (relative to the ground) which is the source of energy from the perspective of the ground. The propeller slows down the wind even when the vehicle itself is moving faster than the wind. The vehicle requires a tail wind (with respect to the ground) in order to operate. As long as there is a tail wind with respect to the ground, then from the vehicle's perspective, the ground is always moving (backwards) faster than the air no matter how fast the vehicle is moving. The drive train uses an effective reduction in gearing, generating greater thrust (force) at lower speed against the relatively slower moving air, than the (opposing) ground force and speed from the relatively faster moving ground, despite energy losses in this conversion.

It is also possible to create a vehicle that moves directly upwind faster than the wind. In this case a turbine drives the wheels, with the reduction in gearing driving the wheels at greater force and lower speed than the turbine.

Practical vehicles

The first known example of such a vehicle is the Bauer cart, created by Andrew Bauer of Douglas Aircraft who built and demonstrated such a vehicle in 1969. Besides still photography, a film has been found showing it in operation.^[54]

However, the basic principle behind DDWFTTW vehicles was utilized as far back as 1877 with the Brennan torpedo, where wires were pulled backwards from spools that drove propellers which in turn drove the torpedo forwards in the water. The wires were unwound from spools on the torpedo and wound onto powered take up spools on the ground. Instead of using land based drums to reel in the wires, consider the case where there is a stream of water, and the wires are attached to non-moving posts. The same basic principle applies to the torpedo, there is effective gearing reduction from wires to the propellers, that results in greater thrust (force) at lower speed, and as long as the water is moving, then a Brennan torpedo can move directly downstream faster than the stream.

Following an internet debate about a brain teaser, a team of students who were unaware of the Bauer cart, conceived and built a wind propeller based land yacht, with the propeller coupled to its wheels through transmission with gears, and filmed it. Due to speculation that the film was a hoax, other teams developed small models that were mostly run on treadmills, but these were still challenged. A full scale vehicle called the Blackbird was then developed in cooperation with the San Jose State University aviation department in a project sponsored by Google.^[55] During the course of this project, Bauer's work from 40 years earlier was rediscovered.^[56]

During the development period, MIT professor Mark Drela published the equations for such a vehicle.^[57] He concluded that it was possible and would not be difficult to create. His equations and conclusions were supported by several others.^{[58][59][60][61][62]} Such a device was built and tested in 2006.^[63]

On July 3, 2010, the propeller-powered land yacht Blackbird set the world's first certified record for going directly downwind, faster than the wind, using only power from the available wind.^[64] The yacht achieved a dead downwind speed of about 2.8 times the speed of the wind.^{[65][66][67][68][69][70]}

See also

• Forces on sails

Notes

1. Jobson, Gary (1990). Championship Tactics: How Anyone Can Sail Faster, Smarter, and Win Races. New York: St. Martin's Press. p. 323. ISBN 0-312-04278-7. ... as the boat speeds up the apparent wind direct goes further and further forward without stalling the sails and the apparent wind speed also increases - so increasing the boat's speed even further. This particular factor is exploited to the full in sand-yachting in which it is common for a sand yacht to exceed the wind speed as measured by a stationary observer.
2. A clear explanation, with diagrams, is given at "The physics of sailing". and at "Physics for Architects: Can Sailboats Sail Faster than the Wind?".
3. An explanation with a video is given at "The Physics of Sailing: Physicists Explain How To Sail Faster than the Wind".
4. A more detailed discussion is given at "The physics of sailing (article published by MIT)" (PDF).
5. A very comprehensive explanation of all aspects of the topic is found in the book: Bethwaite, Frank (2007). High Performance Sailing. Adlard Coles Nautical. ISBN 978-0-7136-6704-2. See in particular Chapter 16.
6. See also the explanation at Terence Tao (March 23, 2009). "Sailing into the wind, or faster than the wind". Retrieved 2010-08-25.
7. A simple explanation is given at "Sailblogs: More on sailing faster than the wind". Sailblogs. September 6, 2008. Retrieved 2010-08-25.
8. How yachts go faster than the wind Gray, R. The Telegraph 26 September 2013
9. "About eXtreme 40". eXtreme40. Retrieved 2010-08-25.
10. "The Winged World of C Cats". Sail Magazine. Archived from the original on March 14, 2010. Retrieved 2010-08-25.
11. See "How fast do these things really go?" in the "Frequently Asked Questions". Four Lakes Ice Yacht Club. Retrieved 2010-08-25.
12. Bob Dill (July 13, 2003). "Frequently Asked Questions". North American Land Sailing Association. Retrieved 2010-08-25.
13. "AC34 Multihull Class Rule Concept Document" (PDF). 34th America's Cup. Retrieved 2010-09-14.
14. "New high performance yachts for 34th America's Cup" (PDF). 34th America's Cup. 2 July 2010. Retrieved 2010-09-14.
15. The monohull concept for the 34th America's Cup called for a design that would achieve 1.0 times true wind speed upwind and 1.4 times downwind, see "AC34 Monohull Class Rule Concept Document" (PDF). 34th America's Cup. Retrieved 2010-09-14.
16. "Emirates Team New Zealand gets leg up on ORACLE TEAM USA". 2012-13 America's Cup Event Authority. 7 September 2013. Retrieved 8 September 2013.
17. The 500-meter record was 51.36 knots (95.12 km/h; 59.10 mph), achieved in 30-knot (56 km/h; 35 mph) winds by Hydroptère, a hydrofoil trimaran, see "Hydroptère World Records". World Sailing Speed Record Council. September 23, 2009. Retrieved 2010-08-25.
18. "Official web site of l'Hydroptère". Retrieved 2010-08-25.
19. The record was 126 mph (109 kn; 203 km/h) with winds of 30–50 mph (48–80 km/h), see Bob Dill (4/5/2009). "Measurement report for Speed Record Attempt Made by Richard Jenkins in the Yacht Greenbird on March 26, 2008". North American Land Sailing Association. Retrieved 2010-08-25. Check date values in: |date= (help)
20. "500 Metre Records". World Sailing Speed Record Council.
21. Forward means making a smaller angle relative to the bow than the angle that the true wind makes relative to the bow. This phenomenon is explained in most sailing manuals, see for example p. 82 of The New Glénans Sailing Manual. David & Charles. 1978. ISBN 0-7153-7470-2. Or see p. 32 and p. 122 of The New Complete Sailing Manual. Dorling Kindersley. 2005. ISBN 978-1-4053-0255-5.
22. An explanation of how this applies to iceboats can be found at the bottom of the "FAQ published by the Four Lakes Ice Yacht Club". Retrieved 2010-08-25.
23. The formula for the apparent wind is (using the symbols shown in the vector diagrams) tan(alpha)=sin(beta)/ (Boat speed+cos(beta)). The figures shown for the angle of the apparent wind assume a boat speed around 0.3 times windspeed, say 6 knots (11 km/h; 6.9 mph) for a keelboat in 18-knot (33 km/h; 21 mph) winds. The figures for the angle of the true wind are from the main article on sailing.
24. "Russell Coutts Talks About BMW Oracle's Giant Multi-hull". cupinfo.com. April 13, 2009. Retrieved 2010-08-25.
25. See also p. 204 of the cited book High Performance Sailing
26. See page 204 of the cited book High Performance Sailing.
27. The maximum multiple of windspeed is achieved at an angle of 90+alpha off the true wind. For alpha = 45, the maximum multiple of windspeed is 1.41 at an angle of 135 degrees off the true wind.
28. See the charts at the end of "the official measurement report". Retrieved 2010-08-25.
29. See section 16.13 and in particular Figure 16.10 of the cited book High Performance Sailing, and more generally Chapter 24
30. See the bottom of the "FAQ published by the Four Lakes Ice Yacht Club". Retrieved 2010-08-25.
31. The values in the table are derived from the formulas shown in the section Vector diagrams and formulas
32. A detailed explanation, with examples, can also be found in Figure 24.2 of the cited book High Performance Sailing
33. If a boat sails at an angle beta to the true wind, then its velocity made good is cos(beta)*boat_speed.
34. Zack Leonard (11-06-2003). "Basic Downwind Performance, Part Two". SailNet. Retrieved 2010-08-25. Check date values in: |date= (help)
35. It should be noted that, in such a situation, the boat is jibing with respect to the true wind, but it is tacking with respect to the apparent wind, because of the apparent wind shift.
36. See Marchaj, Czeslaw Anthony (1979). Aero-hydrodynamics of Sailing. Dodd, Mead & Company, New York. See in particular Chapter I (High speed sailing, pp. 84-127) and Chapter H (Land and hard-water sailing craft, pp. 128-152) of Part 1. The latter contains speed polars along with the equations for maximum speeds and states that it can be expected that the maximum ratio of downwind VMG to true wind would be in the order of 2.1-2.6.
37. "Animation demonstrating the principle of how it can be possible to go faster than the wind".
38. "Dans le mille". Hydroptère. Archived from the original on September 21, 2010. Retrieved 2010-08-25.. Its velocity made good downwind was therefore about equal to the speed of the true wind.
39. Bob Dill (November 2004). "Putting Numbers on Iceboat Performance" (PDF). North American Land Sailing Association. Retrieved 2010-08-25.
40. See in particular pages 3 and 4 of Bob Dill (March 2003). "Sailing Yacht Design for Maximum Speed" (PDF). Scuttlebutt.com. Retrieved 2010-06-21.
41. "Training in Racing Mode". Consorcio Valencia. February 4, 2010. Retrieved 2010-08-25.
42. "Friday the Third, PT 1?". Consorcio Valencia. February 11, 2010. Retrieved 2010-08-25.
43. Pierre Nusslé (February 13, 2010). "La démonstration de puissance d'Oracle brise le rêve d'Alinghi". Tribune de Genève. Retrieved 2010-08-25.
44. "First blood to USA – News – 33rd America's Cup". Consorsio Valencia. 2007-06-25. Retrieved 2010-08-25.
45. "BMW ORACLE Racing Wins Race One". BMW ORACLE Racing. 2003-09-30. Retrieved 2010-08-25.
46. "America's Cup, the numbers of a victory". Yacht Online. Retrieved 2010-03-09.
47. "USA win 33rd America's Cup Match – News – 33rd America's Cup". Consorcio Valencia. Retrieved 2010-08-25.
48. "BMW ORACLE Racing crosses the finish line ahead in Race Two". BMW ORACLE Racing. 2003-09-30. Retrieved 2010-08-25.
49. See minute 30 of podcast interview with USA 17 designer Mike Drummond at "Oracle Racing's USA 17". Omega Tau. 20 July 2011. Retrieved 12 August 2011.; a clear explanation of how the boat can sail downwind at more than twice the speed of the wind is given at minute 59 of the interview.
50. Yoav Raz (April 2009). "Sailboat speed Vs. wind speed". Amateur Yacht Research Society (Catalyst, Journal of the Amateur Yacht Research Society). Retrieved 2010-08-25.
51. A good discussion of polar charts for sailboats can be found at Stan Honey and Jim Teeters (June 10, 2008). "Get Your Performance on Target". Sailing World. Retrieved 2010-08-25.
52. According to the polar chart in section 24.1 (Figure 24.1) of the cited book High Performance Sailing the 18ft Skiff can make good 13 knots downwind in 10 knots of wind and 20 knots in 15 knots of wind.
53. Another good explanation of a polar chart, which indicates that a high-performance boat can make good downwind faster than the wind, is found at page 123 of The New Complete Sailing Manual. Dorling Kindersley. 2005. ISBN 978-1-4053-0255-5.
54. "Andrew Bauer Wind Machine 1969 (no audio)".
55. Adam Fischer (February 28, 2011). "One Man's Quest to Outrace Wind". Wired.
56. Bauer, Andrew (1969). "Faster Than The Wind" (PDF). Marina del Rey, California: First AIAA. Symposium on Sailing., Picture of Bauer with his cart
57. Drela, Mark. "DDFTTW Power Analysis" (PDF). Retrieved June 15, 2010.
58. Boyle, Rebecca (June 2, 2010). "Wind Powered Actually Moves Faster Than Wind Speed, Answering Tricky Physics Question". popsci.com. Retrieved July 1, 2010.
59. Ruina, Andy (1978). "The push-me pull-you boat is better still" (PDF). Retrieved 2012-04-21.
60. Drela, Mark. "Dead-Downwind Faster Than The Wind (DFTTW) Analysis" (PDF). Retrieved June 15, 2010.
61. Gaunaa, Mac; Øye, Stig; Mikkelsen, Robert (2009). "Theory and Design of Flow Driven Vehicles Using Rotors for Energy Conversion". Marseille, France: Proceedings EWEC 2009.
62. "A lecture about upwind-carts & DDWFTTW-carts at the Technical University of Denmark". Youtube. Retrieved 2012-05-02.
63. Goodman, Jack (January 2006). "Down wind faster than the wind" (PDF). Catalyst. Journal of the Amateur Yacht Research Society. Retrieved 2010-09-21.
64. Rick Cavallaro (August 27, 2010). "A Long, Strange, Trip Downwind Faster Than the Wind". Wired. Retrieved 2010-09-14.
65. "Direct Downwind Record Attempts". NALSA. August 2, 2010. Retrieved August 6, 2010.
66. Livingstone, Kimball (August 1, 2010). "A NALSA Record: DDWFTTW". Blue Planet Times. Retrieved August 6, 2010.
67. Livingstone, Kimball (August 2, 2010). "Downwind Noir: The Record". Blue Planet Times. Retrieved August 6, 2010.
68. Cort, Adam (April 5, 2010). "Running Faster than the Wind". sailmagazine.com. Retrieved April 6, 2010.
69. "Ride Like the Wind (only faster)". Retrieved April 6, 2010.
70. Barry, Keith (June 2, 2010). "Wind Powered Car Travels Downwind Faster Than The Wind". wired.com. Retrieved July 1, 2010.

Further reading

• Bethwaite, Frank (1993). High Performance Sailing. Waterline (1993), Thomas Reed Publications (1996, 1998, and 2001), and Adlard Coles Nautical (2003 and 2007). ISBN 978-0-7136-6704-2.

This book provides a comprehensive description of technological developments up to 1993 that have permitted the developments of sailboats that can sail faster than the wind. It covers in particular the 18ft Skiff. It also covers in detail boat and sail handling techniques (course to sail, sail trim, handling waves, etc.) required to reach high speeds. Rod Carr, former British Olympic Sailing Team Manager stated: "[This book] represents a breakthrough in the way it related the theoretical aspects of wind, sea state and rig shape to the way a crew would sail and handle a boat during a race."

External links

• World Sailing Speed Record Council
• North American Land Sailing Association
• The Four Lakes Ice Yacht Club