Waterman butterfly projection

Waterman sphere cluster W5
Waterman polyhedron w5
Waterman projection (Pacific centered)

The Waterman "Butterfly" World Map is a map arrangement created by Steve Waterman. Waterman first published a map in this arrangement in 1996. The arrangement is an unfolding of a globe treated as a truncated octahedron, evoking the butterfly map principle first developed by Bernard J.S. Cahill (1866–1944) in 1909. Cahill and Waterman maps can be shown in various profiles, typically linked at the north Pacific or north Atlantic oceans.

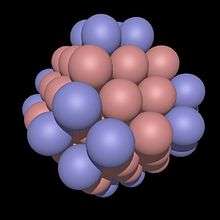
As Cahill was an architect, his approach tended toward forms that could be demonstrated physically, such as by his flattenable rubber-ball map. Waterman, on the other hand, derived his design from his work on close-packing of spheres. This involves connecting the sphere centers from cubic closest-packed spheres into a corresponding convex hull, as demonstrated in the accompanying graphics. These illustrate the W5 sphere cluster, W5 convex hull, and two Waterman projections from the W5 convex hull.

To project the polyhedron to the plane, straight lines are used to define each 5 × 5 section onto this convex hull. According to annotations on modern versions of the map, the projection divides the equator equally amongst the meridians. Popko notes the projection can be gnomonic too.[1] The two methods yield very similar results. Parallels of latitude are drawn as three straight-line sections in each octant: from pole to fold-line; from fold-line to longest line parallel to equator; and then to the equator. The longest line parallel to the equator also has equal-length delineations. Waterman chose a specific Waterman polyhedron and central meridian to minimize interrupting major land masses.

Like Buckminster Fuller's 1943 Dymaxion Projection, an octahedral butterfly map can show all the continents uninterrupted if its octants are divided at a suitable meridian (in this case 20°W) and are joined, for example, at the North Atlantic, as in the 1996 version.[2][3]

See also

References

  1. ↑ Edward S., Popko (2012). Divided Spheres: Geodesics and the Orderly Subdivision of the Sphere. Taylor & Francis. pp. 20–21. ISBN 9781466504295.
  2. ↑ Darvias, Gyorgy (2002). Symmetry: Culture and Science. Symmetrion. pp. 129–171. ISBN 963 214 761 8.
  3. ↑ Dongo, Studio (2013). The City That Traveled The World. CreateSpace Independent Publishing Platform. pp. cover and acknowledgements page. ISBN 9781484966228.
This article is issued from Wikipedia - version of the 12/2/2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.